Solutions to Linear Algebra, Stephen H. Friedberg, Fourth Edition (Chapter 4)

Solution maual to Linear Algebra, Fourth Edition, Stephen H. Friedberg. (Chapter 4)

Solutions to Linear Algebra, Fourth Edition, Stephen H. Friedberg. (Chapter 4)

Linear Algebra solution manual, Fourth Edition, Stephen H. Friedberg. (Chapter 4)

Linear Algebra solutions Friedberg. (Chapter 4)

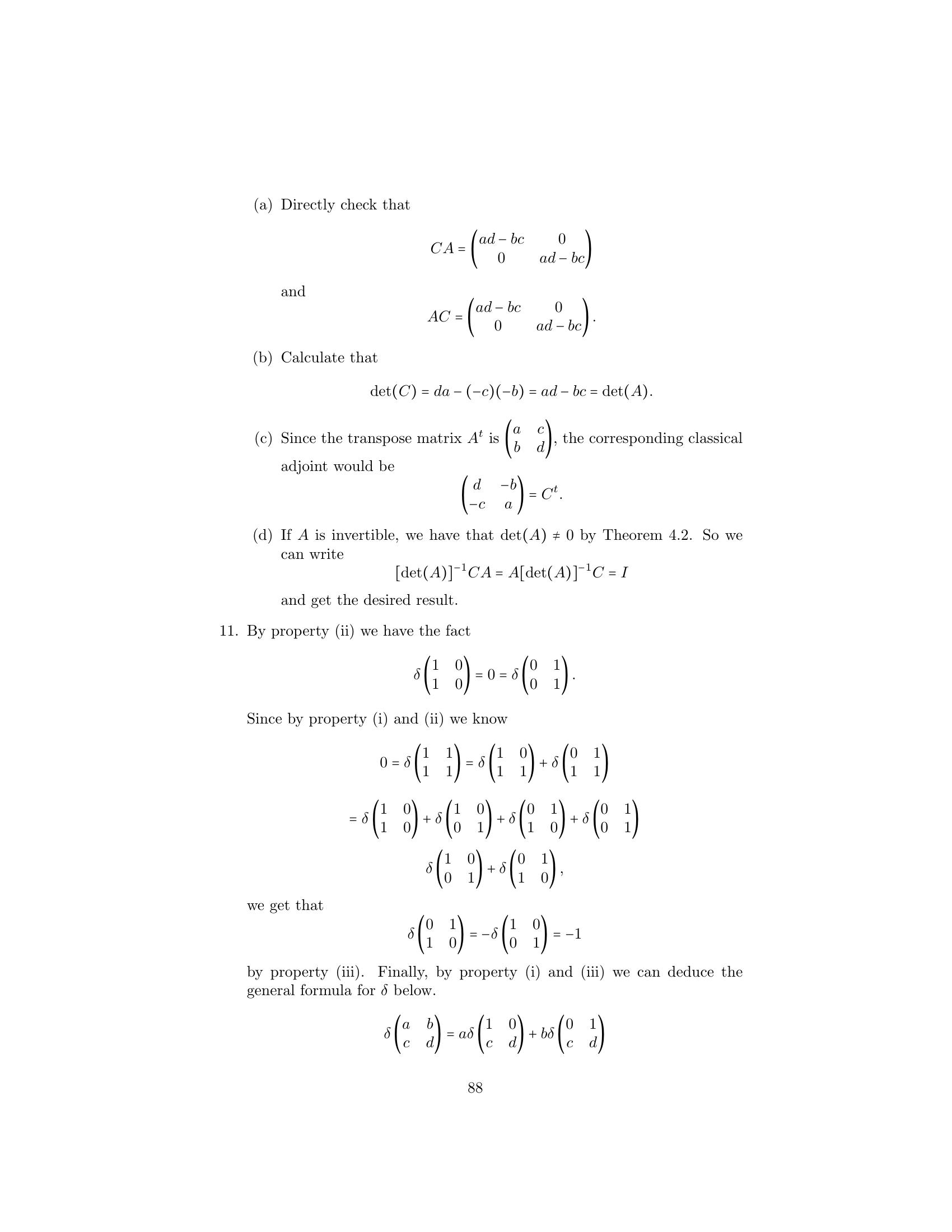
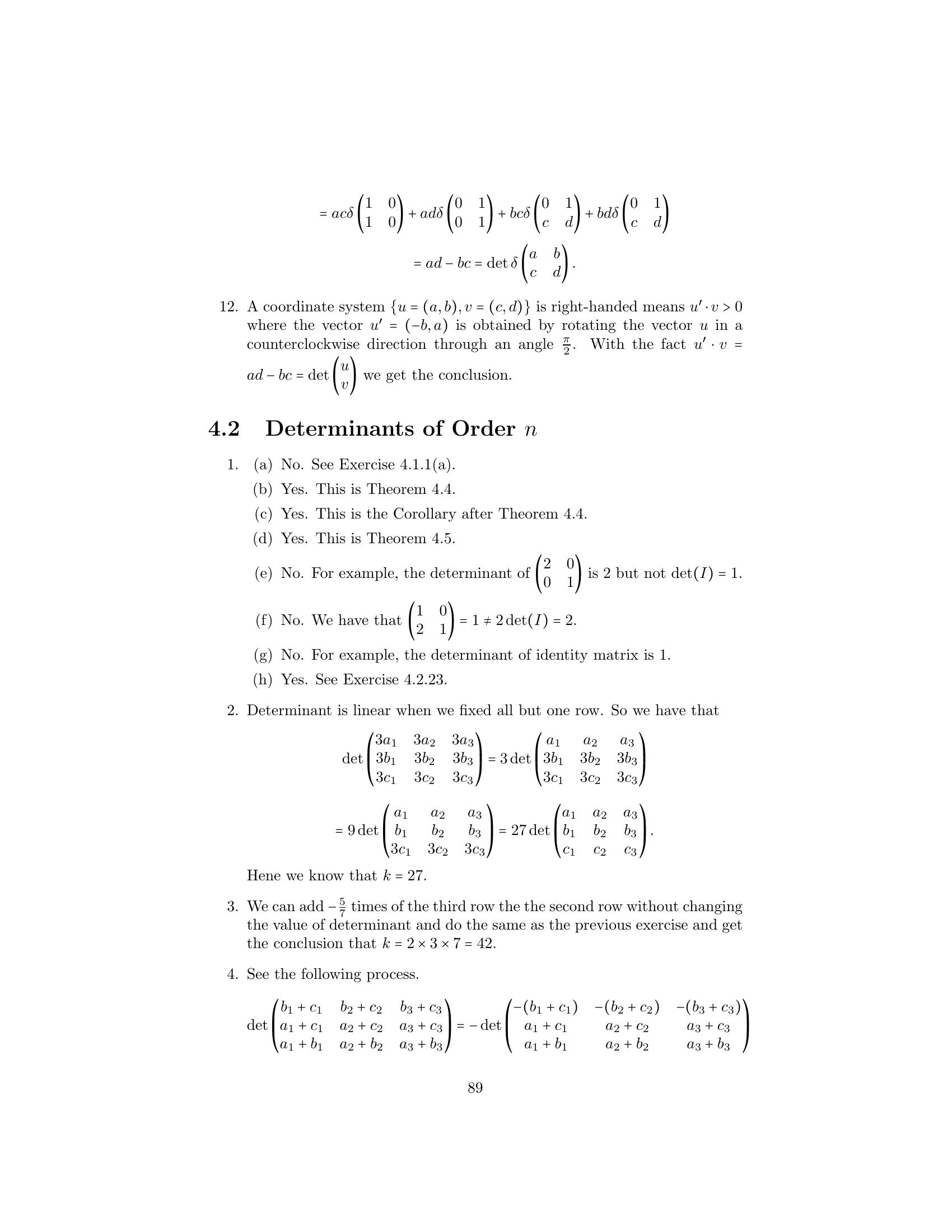
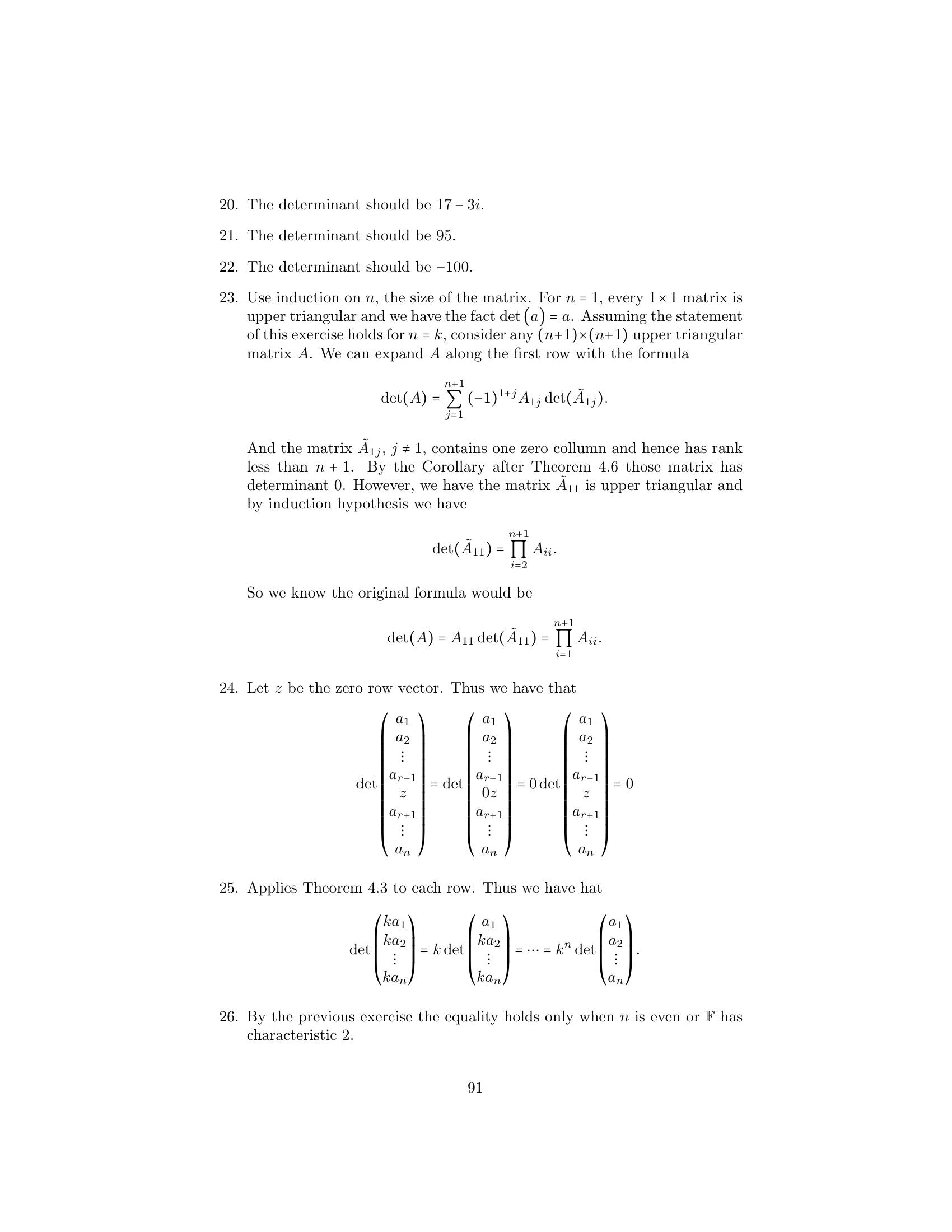
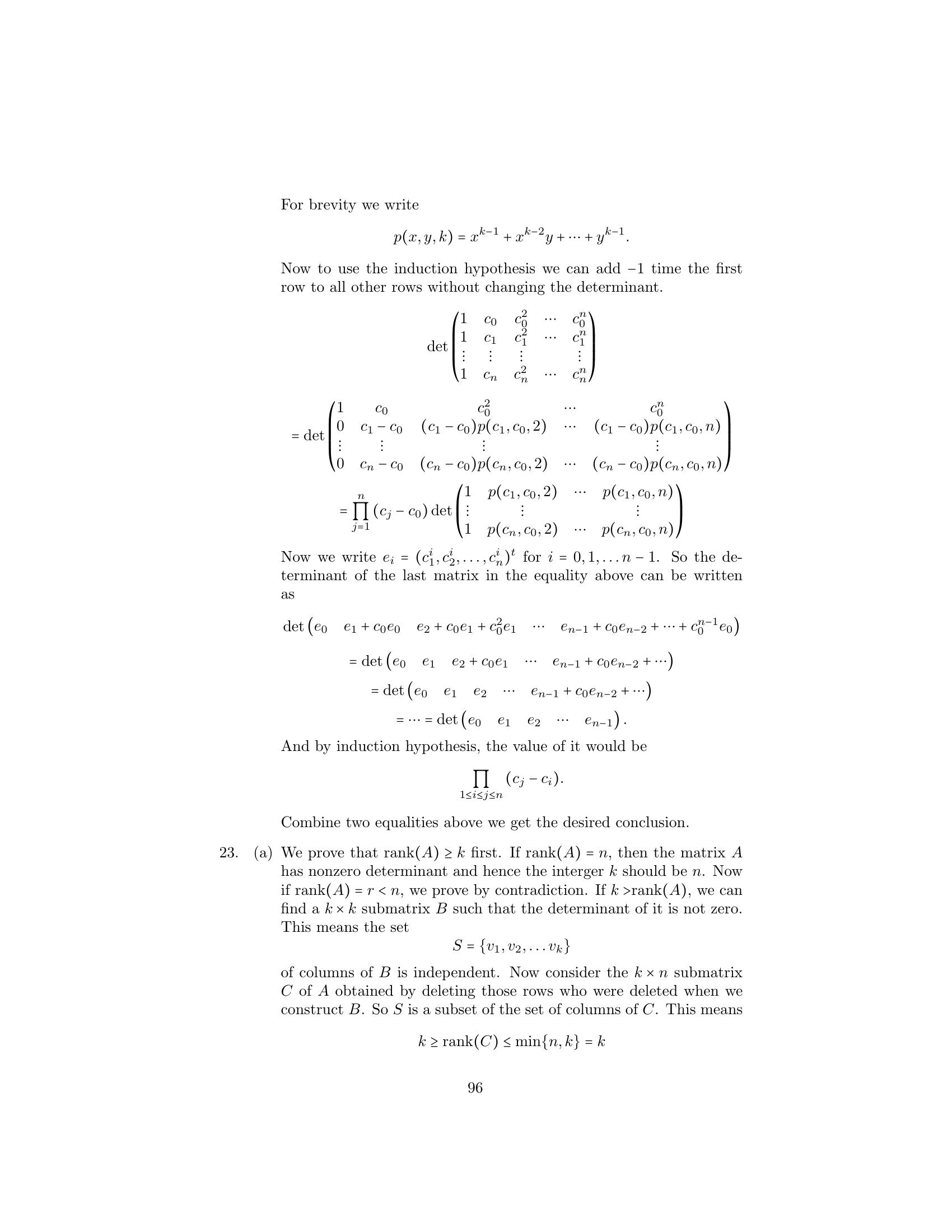
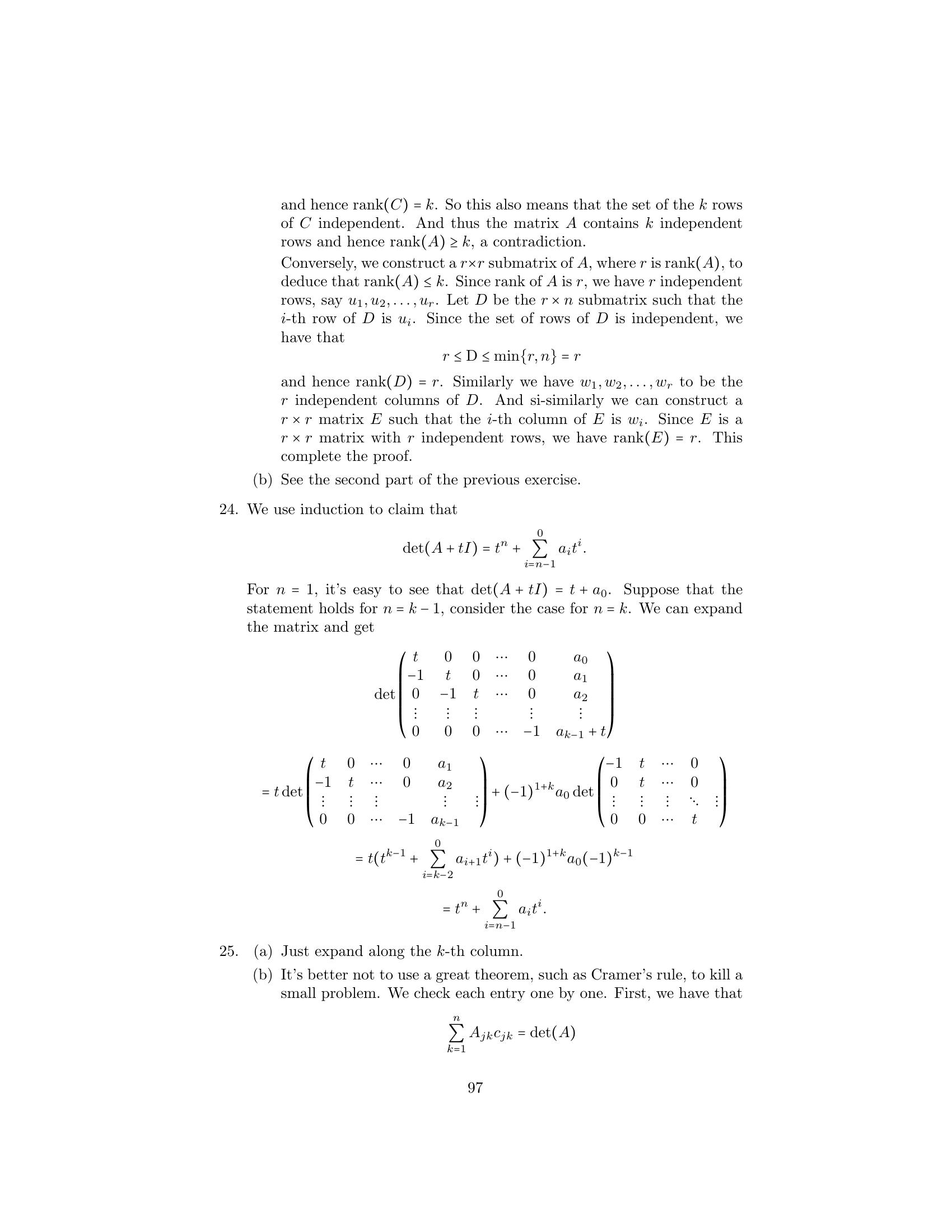
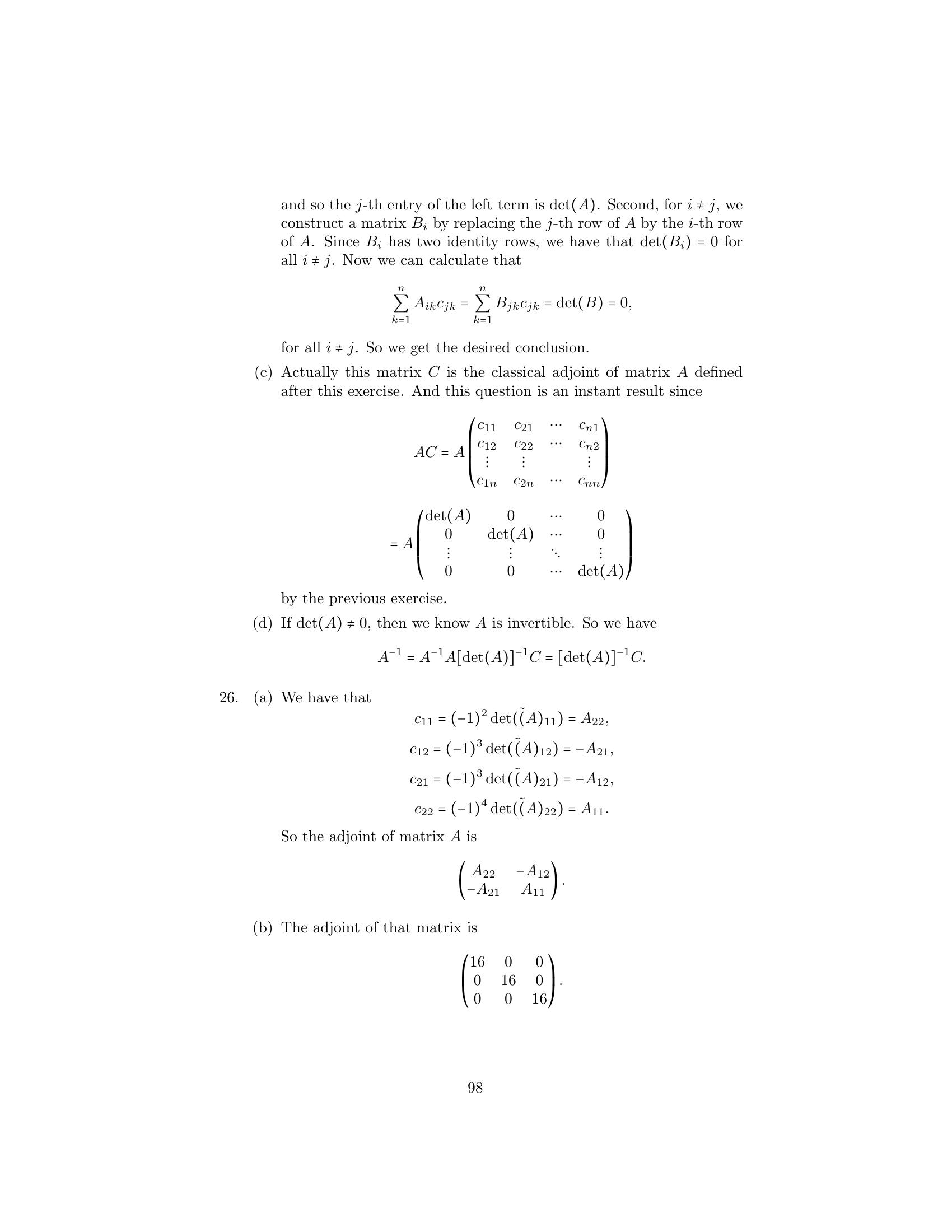
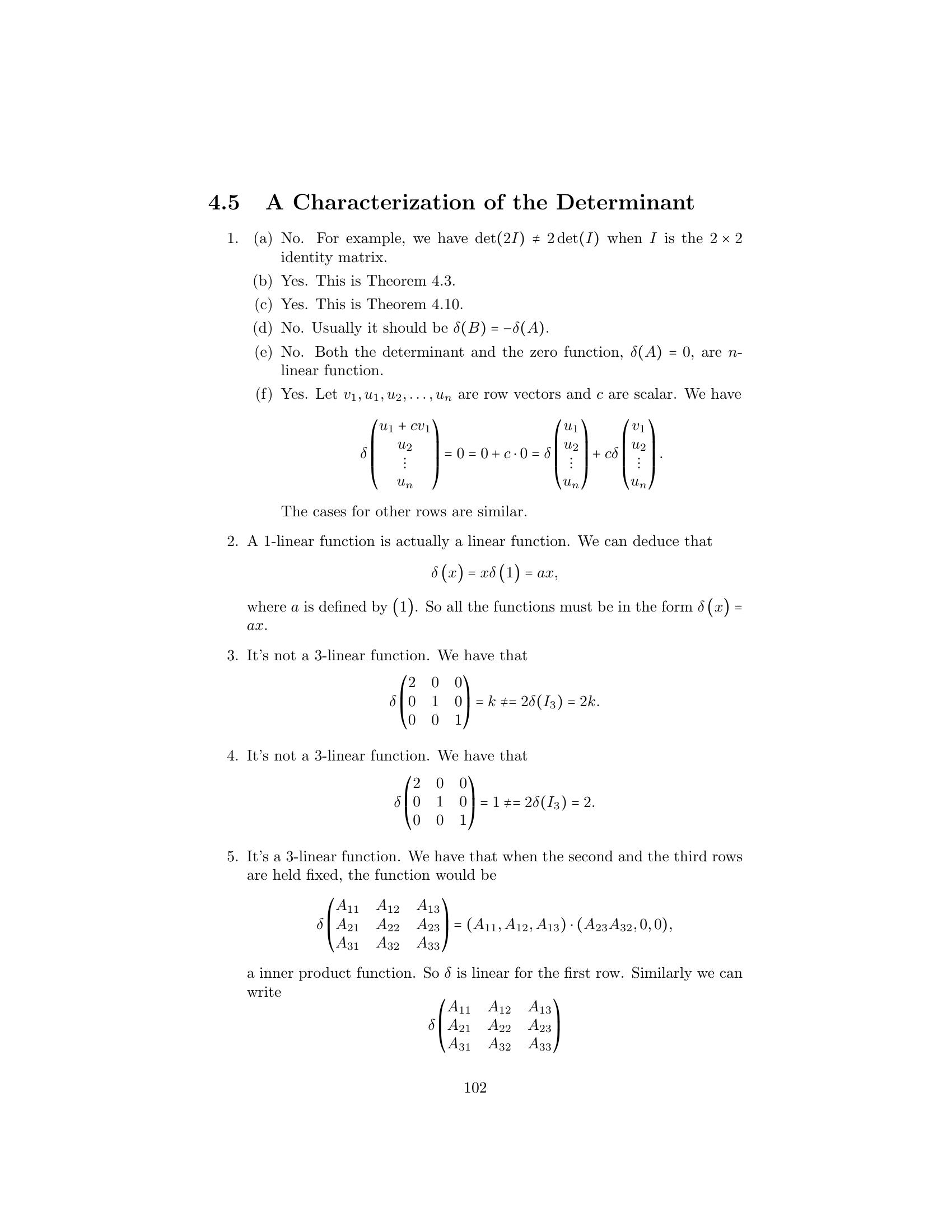
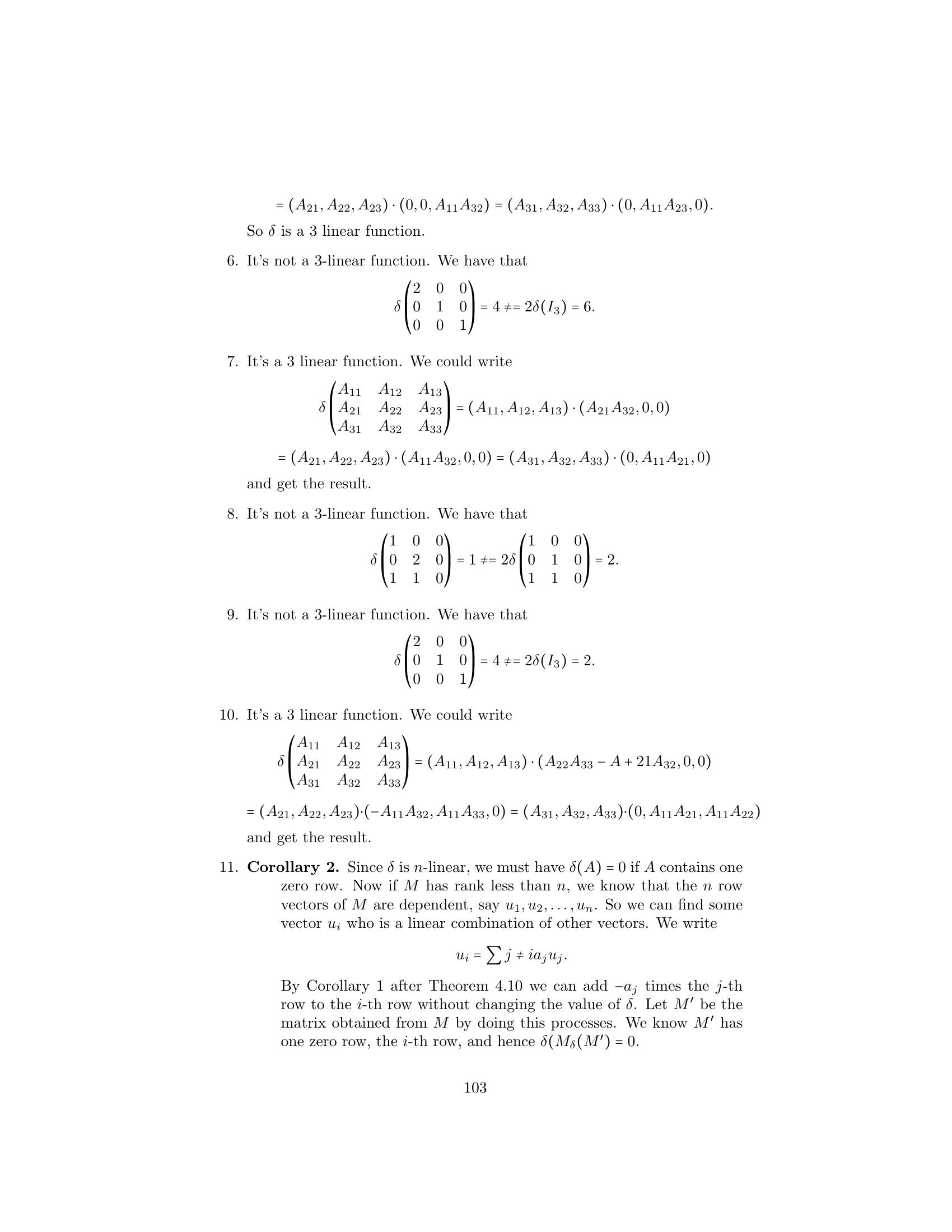
1.Label the following statements as true or false. (a) The function det : M2×2 (F ) → F is a linear transformation. (b) The determinant of a 2 × 2 matrix is a linear function of each row of the matrix when the other row is held fixed. (c) If A ∈ M2×2 (F ) and det(A) = 0, then A is invertible. (d) If u and v are vectors in R2 emanating from the origin, then the area of the parallelogram having u and v as adjacent sides is  u det . v 208 Chap. 4 Determinants (e) A coordinate system is right-handed if and only if its orientation equals 1. 2. Compute the determinants of the following matrices in M2×2 (R).    6 −3 −5 2 8 0 (a) (b) (c) 2 4 6 1 3 −1 3. Compute the determinants of the following matrices in M2×2 (C).    −1 + i 1 − 4i 5 − 2i 6 + 4i 2i 3 (a) (b) (c) 3 + 2i 2 − 3i −3 + i 7i 4 6i 4. For each of the following pairs of vectors u and v in R2 , compute the area of the parallelogram determined by u and v. (a) u = (3, −2) and v = (2, 5) (b) u = (1, 3) and v = (−3, 1) (c) u = (4, −1) and v = (−6, −2) (d) u = (3, 4) and v = (2, −6) 5. Prove that if B is the matrix obtained by interchanging the rows of a 2 × 2 matrix A, then det(B) = − det(A). 6. Prove that if the two columns of A ∈ M2×2 (F ) are identical, then det(A) = 0. 7. Prove that det(At ) = det(A) for any A ∈ M2×2 (F ). 8. Prove that if A ∈ M2×2 (F ) is upper triangular, then det(A) equals the product of the diagonal entries of A. 9. Prove that det(AB) = det(A)· det(B) for any A, B ∈ M2×2 (F ). 10. The classical adjoint of a 2 × 2 matrix A ∈ M2×2 (F ) is the matrix  A22 −A12 C = . −A21 A11 Prove that (a) CA = AC = [det(A)]I. (b) det(C) = det(A). (c) The classical adjoint of At is C t . (d) If A is invertible, then A−1 = [det(A)]−1 C. 11. Let δ : M2×2 (F ) → F be a function with the following three properties. (i) δ is a linear function of each row of the matrix when the other row is held fixed. (ii) If the two rows of A ∈ M2×2 (F ) are identical, then δ(A) = 0. Sec. 4.2 Determinants of Order n 209 (iii) If I is the 2 × 2 identity matrix, then δ(I) = 1. Prove that δ(A) = det(A) for all A ∈ M2×2 (F ). (This result is general- ized in Section 4.5.) 12.Let {u, v} be an ordered basis for R2 . Prove that  u O =1 v if and only if {u, v} forms a right-handed coordinate system. Hint: Recall the definition of a rotation given in Example 2 of Section 2.1. 1.Label(a)(b)(c)(d)(e)(f )(g)(h)the following statements as true or false. The function det : Mn×n (F ) → F is a linear transformation. The determinant of a square matrix can be evaluated by cofactor expansion along any row. If two rows of a square matrix A are identical, then det(A) = 0. If B is a matrix obtained from a square matrix A by interchanging any two rows, then det(B) = − det(A). If B is a matrix obtained from a square matrix A by multiplying a row of A by a scalar, then det(B) = det(A). If B is a matrix obtained from a square matrix A by adding k times row i to row j, then det(B) = k det(A). If A ∈ Mn×n (F ) has rank n, then det(A) = 0. The determinant of an upper triangular matrix equals the product of its diagonal entries. Sec. 4.2 Determinants of Order n 2. Find the value of k that satisfies the following equation: ⎛ ⎞ ⎛ ⎞ 3a1 3a2 3a3 a1 a2 a3 det ⎝ 3b1 3b2 3b3 ⎠ = k det ⎝ b1 b2 b3 ⎠ . 3c1 3c2 3c3 c1 c2 c3 221 3. Find the value of k that satisfies the following equation: ⎛ ⎞ ⎛ ⎞ 2a1 2a2 2a3 a1 a2 a3 det ⎝3b1 + 5c1 3b2 + 5c2 3b3 + 5c3 ⎠ = k det ⎝ b1 b2 b3 ⎠ . 7c1 7c2 7c3 c1 c2 c3 4. Find the value of k that satisfies the following equation: ⎛ ⎞ ⎛ ⎞ b1 + c1 b2 + c2 b3 + c3 a1 a2 a3 det ⎝a1 + c1 a2 + c2 a3 + c3 ⎠ = k det ⎝ b1 b2 b3⎠ . a1 + b1 a2 + b2 a3 + b3 c1 c2 c3 In Exercises 5–12, evaluate the determinant of the given matrix by cofactor expansion along the indicated row. ⎛ ⎞ ⎛ ⎞ 0 1 2 1 0 2 ⎝−1 0 −3⎠ ⎝ 0 1 5⎠ 5. 6. 2 3 0 −1 3 0 along the first row along the first row ⎛ ⎞ ⎛ ⎞ 0 1 2 1 0 2 ⎝−1 0 −3⎠ ⎝ 0 1 5⎠ 7. 8. 2 3 0 −1 3 0 along the second row along the third row ⎛ ⎞ ⎛ ⎞ 0 1+ i 2 i 2+ i 0 ⎝−2i 0 1 − i⎠ ⎝−1 3 2i ⎠ 9. 10. 3 4i 0 0 −1 1 − i along the third row along the second row ⎛ ⎞ ⎛ ⎞ 0 2 1 3 1 −1 2 −1 ⎜ ⎜ 1 0 −2 2⎟ ⎟ ⎜−3 ⎜ 4 1 −1⎟ ⎟ 11. ⎝ 3 −1 0 1⎠ 12. ⎝ 2 −5 −3 8⎠ −1 1 2 0 −2 6 −4 1 along the fourth row along the fourth row In Exercises 13–22, evaluate the determinant of the given matrix by any le- gitimate method. 222 Chap. 4 Determinants ⎛ ⎞ ⎛ ⎞ 0 0 1 2 3 4 13. ⎝0 2 3⎠ 14. ⎝5 6 0⎠ 4 5 6 7 0 0 ⎛ ⎞ ⎛ ⎞ 1 2 3 −1 3 2 15. ⎝4 5 6⎠ 16. ⎝ 4 −8 1⎠ 7 8 9 2 2 5 ⎛ ⎞ ⎛ ⎞ 0 1 1 1 −2 3 17. ⎝ 1 2 −5⎠ 18. ⎝−1 2 −5⎠ 6 −4 3 3 −1 2 ⎛ ⎞ ⎛ ⎞ i 2 −1 −1 2 + i 3 19. ⎝ 3 1+ i 2 ⎠ 20. ⎝1 − i i 1 ⎠ −2i 1 4 − i 3i 2 −1 + i ⎛ ⎞ ⎛ ⎞ 1 0 −2 3 1 −2 3 −12 21. ⎜−3 ⎜ 1 1 2⎟ ⎟ 22. ⎜−5 ⎜ 12 −14 19⎟ ⎟ ⎝ 0 4 −1 1⎠ ⎝−9 22 −20 31⎠ 2 3 0 1 −4 9 −14 15 23. Prove that the determinant of an upper triangular matrix is the product of its diagonal entries. 24. Prove the corollary to Theorem 4.3. 25. Prove that det(kA) = kn det(A) for any A ∈ Mn×n (F ). 26. Let A ∈ Mn×n (F ). Under what conditions is det(−A) = det(A)? 27. Prove that if A ∈ Mn×n (F ) has two identical columns, then det(A) = 0. 28. Compute det(Ei ) if Ei is an elementary matrix of type i. 29. † Prove that if E is an elementary matrix, then det(E t ) = det(E). 30. Let the rows of A ∈ Mn×n (F ) be a1 , a2 , . . . , an , and let B be the matrix in which the rows are an , an−1 , . . . , a1 . Calculate det(B) in terms of det(A). 1.Label the following statements as true or false. (a) (b) (c) (d) (e) (f ) (g)(h)If E is an elementary matrix, then det(E) = ±1. For any A, B ∈ Mn×n(F ), det(AB) = det(A)· det(B). A matrix M ∈ Mn×n (F ) is invertible if and only if det(M ) = 0. A matrix M ∈ Mn×n (F ) has rank n if and only if det(M ) = 0. For any A ∈ Mn×n (F ), det(At ) = − det(A). The determinant of a square matrix can be evaluated by cofactor expansion along any column. Every system of n linear equations in n unknowns can be solved by Cramer’s rule. Let Ax = b be the matrix form of a system of n linear equations in n unknowns, where x = (x1 , x2 , . . . , xn )t . If det(A) = 0 and if Mk is the n × n matrix obtained from A by replacing row k of A by bt , then the unique solution of Ax = b is xk = det(Mk ) det(A) for k = 1, 2, . . . , n. In Exercises 2–7, use Cramer’s rule to solve the given system of linear equa- tions. 2. a11 x1 + a12 x2 = b1 a21 x1 + a22 x2 = b2 where a11 a22 − a12 a21 = 0 2x1 + x2 − 3x3 = 5 3. x1 − 2x2 + x3 = 10 3x1 + 4x2 − 2x3 = 0 2x1 + x2 − 3x3 = 1 4. x1 − 2x2 + x3 = 0 3x1 + 4x2 − 2x3 = −5 x1 − x2 + 4x3 = −2 6. −8x1 + 3x2 + x3 = 0 2x1 − x2 + x3 = 6 x1 − x2 + 4x3 = −4 5. −8x1 + 3x2 + x3 = 8 2x1 − x2 + x3 = 0 3x1 + x2 + x3 = 4 7. −2x1 − x2 = 12 x1 + 2x2 + x3 = −8 8.Use Theorem 4.8 to prove a result analogous to Theorem 4.3 (p. 212), but for columns. 9.Prove that an upper triangular n × n matrix is invertible if and only if all its diagonal entries are nonzero. Sec. 4.3 Properties of Determinants 229 10.11.12.13.14.15.16.17.†A matrix M ∈ Mn×n (C) is called nilpotent if, for some positive integer k, M k = O, where O is the n × n zero matrix. Prove that if M is nilpotent, then det(M ) = 0. A matrix M ∈ Mn×n (C) is called skew-symmetric if M t = −M . Prove that if M is skew-symmetric and n is odd, then M is not invert- ible. What happens if n is even? A matrix Q ∈ Mn×n (R) is called orthogonal if QQt = I. Prove that if Q is orthogonal, then det(Q) = ±1. For M ∈ Mn×n (C), let M be the matrix such that (M )ij = Mij for all i, j, where Mij is the complex conjugate of Mij . (a) Prove that det(M ) = det(M ). (b) A matrix Q ∈ Mn×n (C) is called unitary if QQ ∗ = I, where Q∗ = Qt. Prove that if Q is a unitary matrix, then | det(Q)| = 1. Let β = {u1 , u2 , . . . , un } be a subset of Fn containing n distinct vectors, and let B be the matrix in Mn×n (F ) having uj as column j. Prove that β is a basis for Fn if and only if det(B) = 0. Prove that if A, B ∈ Mn×n (F ) are similar, then det(A) = det(B). Use determinants to prove that if A, B ∈ Mn×n (F ) are such that AB = I, then A is invertible (and hence B = A−1 ). Let A, B ∈ Mn×n (F ) be such that AB = −BA. Prove that if n is odd and F is not a field of characteristic two, then A or B is not invertible. 18.19.20.Complete the proof of Theorem 4.7 by showing that if A is an elementary matrix of type 2 or type 3, then det(AB) = det(A)· det(B). A matrix A ∈ Mn×n (F ) is called lower triangular if Aij = 0 for 1 ≤ i < j ≤ n. Suppose that A is a lower triangular matrix. Describe det(A) in terms of the entries of A. Suppose that M ∈ Mn×n (F ) can be written in the form  A B M = , O I where A is a square matrix. Prove that det(M ) = det(A). 21. † Prove that if M ∈ Mn×n (F ) can be written in the form  A B M = , O C where A and C are square matrices, then det(M ) = det(A)· det(C). 230 Chap. 4 Determinants 22. Let T : Pn (F ) → Fn+1 be the linear transformation defined in Exer- cise 22 of Section 2.4 by T(f ) = (f (c0 ), f (c1 ), . . . , f (cn )), where c0 , c1 , . . . , cn are distinct scalars in an infinite field F . Let β be the standard ordered basis for Pn (F ) and γ be the standard ordered basis for Fn+1 . (a) Show that M = [T]γβ has the form ⎛ ⎞ 1 c0 c20 · · · cn 0 ⎜1 ⎜ c1 c21 · · · cn 1 ⎟ ⎟ ⎜ . ⎟ . ⎝ .. .. . .. . .. . ⎠ 1 cn c2 n · · · cnn (b)(c)A matrix with this form is called a Vandermonde matrix. Use Exercise 22 of Section 2.4 to prove that det(M ) = 0. Prove that  det(M ) = (cj − ci ), 0≤i<j≤n the product of all terms of the form cj − ci for 0 ≤ i < j ≤ n. 23. Let A ∈ Mn×n (F ) be nonzero. For any m (1 ≤ m ≤ n), an m × m submatrix is obtained by deleting any n − m rows and any n − m columns of A. (a) Let k (1 ≤ k ≤ n) denote the largest integer such that some k × k submatrix has a nonzero determinant. Prove that rank(A) = k. (b) Conversely, suppose that rank(A) = k. Prove that there exists a k × k submatrix with a nonzero determinant. 24. Let A ∈ Mn×n (F ) have the form ⎛ ⎞ 0 0 0 ··· 0 a0 ⎜−1 ⎜ 0 0 ··· 0 a1 ⎟ ⎟ A = ⎜ ⎜ 0 −1 0 ··· 0 a2 ⎟ ⎟ . ⎜ ⎝ .. . .. . .. . .. . .. . ⎟ ⎠ 0 0 0 ··· −1 an−1 Compute det(A + tI), where I is the n × n identity matrix. 25.Let cjk denote the cofactor of the row j, column k entry of the matrix A ∈ Mn×n (F ). (a) Prove that if B is the matrix obtained from A by replacing column k by ej , then det(B) = cjk . Sec. 4.3 Properties of Determinants 231 (b) Show that for 1 ≤ j ≤ n, we have ⎛ ⎞ cj1 ⎜ cj2 ⎟ ⎜ ⎟ A ⎜ . ⎟ = det(A)· ej . ⎝ .. ⎠ cjn Hint: Apply Cramer’s rule to Ax = ej . (c) Deduce that if C is the n × n matrix such that Cij = cji , then AC = [det(A)]I. (d) Show that if det(A) = 0, then A−1 = [det(A)]−1 C. The following definition is used in Exercises 26–27. Definition. The classical adjoint of a square matrix A is the transpose of the matrix whose ij-entry is the ij-cofactor of A. 26. Find the classical adjoint of each of the following matrices. ⎛ ⎞  4 0 0 (a) A11 A12 (b) ⎝0 4 0⎠ A21 A22 0 0 4 ⎛ ⎞ ⎛ ⎞ −4 0 0 3 6 7 (c) ⎝ 0 2 0⎠ (d) ⎝0 4 8⎠ 0 0 5 0 0 5 ⎛ ⎞ ⎛ ⎞ 1 − i 0 0 7 1 4 (e) ⎝ 4 3i 0 ⎠ (f ) ⎝ 6 −3 0⎠ 2i 1 + 4i −1 −3 5 −2 ⎛ ⎞ ⎛ ⎞ −1 2 5 3 2+ i 0 (g) ⎝ 8 0 −3⎠ (h) ⎝−1 + i 0 i ⎠ 4 6 1 0 1 3 − 2i 27. Let C be the classical adjoint of A ∈ Mn×n (F ). Prove the following statements. (a) det(C) = [det(A)]n−1 . (b) C t is the classical adjoint of At . (c) If A is an invertible upper triangular matrix, then C and A−1 are both upper triangular matrices. 28. Let y1 , y2 , . . . , yn be linearly independent functions in C∞ . For each y ∈ C∞ , define T(y) ∈ C∞ by ⎛ ⎞ y(t) y1 (t) y2 (t) · · · yn (t) ⎜ y (t) y1  (t) y2  (t) · · · yn  (t) ⎟ ⎜ ⎟ [T(y)](t) = det ⎜ .. .. .. .. ⎟ . ⎝ . . . . ⎠ y (n) (t) y1 (n) (t) y2 (n) (t) · · · yn (n) (t) 232 Chap. 4 Determinants The preceding determinant is called the Wronskian of y, y1 , . . . , yn . (a) Prove that T : C∞ → C∞ is a linear transformation. (b) Prove that N(T) = span({y1 , y2 , . . . , yn }). 1. Label the following statements as true or false. (a)(b)(c)(d)(e)(f )(g)(h)(i)(j)(k)The determinant of a square matrix may be computed by expand- ing the matrix along any row or column. In evaluating the determinant of a matrix, it is wise to expand along a row or column containing the largest number of zero en- tries. If two rows or columns of A are identical, then det(A) = 0. If B is a matrix obtained by interchanging two rows or two columns of A, then det(B) = det(A). If B is a matrix obtained by multiplying each entry of some row or column of A by a scalar, then det(B) = det(A). If B is a matrix obtained from A by adding a multiple of some row to a different row, then det(B) = det(A). The determinant of an upper triangular n×n matrix is the product of its diagonal entries. For every A ∈ Mn×n (F ), det(At ) = − det(A). If A, B ∈ Mn×n (F ), then det(AB) = det(A)· det(B). If Q is an invertible matrix, then det(Q−1 ) = [det(Q)]−1 . A matrix Q is invertible if and only if det(Q) = 0. 2. Evaluate the determinant of the following 2 × 2 matrices.   4 −5 −1 7 (a) (b) 2 3 3 8   2 + i −1 + 3i 3 4i (c) (d) 1 − 2i 3 − i −6i 2i 3.Evaluate the determinant of the following matrices in the manner indi- cated. Sec. 4.4 Summary—Important Facts about Determinants 237 ⎛ ⎞ ⎛ ⎞ 0 1 2 1 0 2 ⎝−1 0 −3⎠ ⎝ 0 1 5⎠ (a) (b) 2 3 0 −1 3 0 along the first row along the first column ⎛ ⎞ ⎛ ⎞ 0 1 2 1 0 2 ⎝−1 0 −3⎠ ⎝ 0 1 5⎠ (c) (d) 2 3 0 −1 3 0 along the second column along the third row ⎛ ⎞ ⎛ ⎞ 0 1+ i 2 i 2+ i 0 ⎝−2i 0 1 − i⎠ ⎝−1 3 2i ⎠ (e) (f ) 3 4i 0 0 −1 1 − i along the third row along the third column ⎛ ⎞ ⎛ ⎞ 0 2 1 3 1 −1 2 −1 ⎜ ⎜ 1 0 −2 2⎟ ⎟ ⎜−3 ⎜ 4 1 −1⎟ ⎟ (g) ⎝ 3 −1 0 1⎠ (h) ⎝ 2 −5 −3 8⎠ −1 1 2 0 −2 6 −4 1 along the fourth column along the fourth row 4. Evaluate the determinant of the following matrices by any legitimate method. ⎛ ⎞ ⎛ ⎞ 1 2 3 −1 3 2 (a) ⎝4 5 6⎠ (b) ⎝ 4 −8 1⎠ 7 8 9 2 2 5 ⎛ ⎞ ⎛ ⎞ 0 1 1 1 −2 3 (c) ⎝1 2 −5⎠ (d) ⎝−1 2 −5⎠ 6 −4 3 3 −1 2 ⎛ ⎞ ⎛ ⎞ i 2 −1 −1 2+ i 3 (e) ⎝ 3 1+ i 2 ⎠ (f ) ⎝1 − i i 1 ⎠ −2i 1 4 − i 3i 2 −1 + i ⎛ ⎞ ⎛ ⎞ 1 0 −2 3 1 −2 3 −12 (g) ⎜−3 ⎜ 1 1 2⎟ ⎟ (h) ⎜−5 ⎜ 12 −14 19⎟ ⎟ ⎝ 0 4 −1 1⎠ ⎝−9 22 −20 31⎠ 2 3 0 1 −4 9 −14 15 5. Suppose that M ∈ M n×n (F ) can be written in the form  A B M = , O I where A is a square matrix. Prove that det(M ) = det(A). 238 Chap. 4 Determinants 6. † Prove that if M ∈ Mn×n (F ) can be written in the form  A B M = , O C where A and C are square matrices, then det(M ) = det(A)· det(C). 1.Label the following statements as true or false. (a)(b)(c)(d)(e)(f )Any n-linear function δ : Mn×n (F ) → F is a linear transformation. Any n-linear function δ : Mn×n (F ) → F is a linear function of each row of an n × n matrix when the other n − 1 rows are held fixed. If δ : Mn×n (F ) → F is an alternating n-linear function and the matrix A ∈ Mn×n (F ) has two identical rows, then δ(A) = 0. If δ : Mn×n (F ) → F is an alternating n-linear function and B is obtained from A ∈ Mn×n (F ) by interchanging two rows of A, then δ(B) = δ(A). There is a unique alternating n-linear function δ : Mn×n (F ) → F . The function δ : Mn×n (F ) → F defined by δ(A) = 0 for every A ∈ Mn×n (F ) is an alternating n-linear function. 2. Determine all the 1-linear functions δ : M1×1 (F ) → F . Determine which of the functions δ : M3×3 (F ) → F in Exercises 3–10 are 3-linear functions. Justify each answer. Sec. 4.5 A Characterization of the Determinant 243 3.4.5.6.7.8.9.10.11.12.13.14.15.16.17.18.19.20.δ(A) = k, where k is any nonzero scalar δ(A) = A22 δ(A) = A11 A23 A32 δ(A) = A11 + A23 + A32 δ(A) = A11 A21 A32 δ(A) = A11 A31 A32 δ(A) = A211 A222 A233 δ(A) = A11 A22 A33 − A11 A21 A32 Prove Corollaries 2 and 3 of Theorem 4.10. Prove Theorem 4.11. Prove that det : M2×2 (F ) → F is a 2-linear function of the columns of a matrix. Let a, b, c, d ∈ F . Prove that the function δ : M2×2 (F ) → F defined by δ(A) = A11 A22 a + A11 A21 b + A12 A22 c + A12 A21 d is a 2-linear function. Prove that δ : M2×2 (F ) → F is a 2-linear function if and only if it has the form δ(A) = A11 A22 a + A11 A21 b + A12 A22 c + A12 A21 d for some scalars a, b, c, d ∈ F . Prove that if δ : Mn×n (F ) → F is an alternating n-linear function, then there exists a scalar k such that δ(A) = k det(A) for all A ∈ Mn×n (F ). Prove that a linear combination of two n-linear functions is an n-linear function, where the sum and scalar product of n-linear functions are as defined in Example 3 of Section 1.2 (p. 9). Prove that the set of all n-linear functions over a field F is a vector space over F under the operations of function addition and scalar mul- tiplication as defined in Example 3 of Section 1.2 (p. 9). Let δ : Mn×n (F ) → F be an n-linear function and F a field that does not have characteristic two. Prove that if δ(B) = −δ(A) whenever B is obtained from A ∈ Mn×n (F ) by interchanging any two rows of A, then δ(M ) = 0 whenever M ∈ Mn×n (F ) has two identical rows. Give an example to show that the implication in Exercise 19 need not hold if F has characteristic two.